Search

What Is Cymatics?

Cymatics is a term that is gaining a lot of popularity in pop culture, and some folks may not fully understand the basic concepts behind the phenomenon. We hope to bridge that gap with this page of definitions and main concepts, as well as a few extra references for further study. At the bottom of the page you will find a selection of videos that further demonstrates the science of cymatics.

Definitions of Key Terms

  1. Cymatics -  The study of wave phenomena and the process of manipulating matter with energy waves (sound or otherwise).
  2. Frequency - The rate at which a pattern occurs over time, measured in Hertz (Hz) where 1Hz is one cycle of the pattern per one second; 20Hz is 20 cycles of a pattern per one second.
  3. Waveform - A mathematical curve, line or graph that depicts the shape of a wave signal. A sine wave is the general wave we usually consider, whereas a triangle wave is basically a sine wave straightened out into lines meeting at triangular points.
  4. Resonance/Resonant - The phenomenon when two or more signals interact with constructive interference; in other words, the signals are synchronized in some manner. When two or more signals are thusly synchronized, they are said to be resonant. A fundamental resonant frequency is the lowest frequency that resonates with a system; it is otherwise called the fundamental tone.
  5. Standing Wave - A state of vibration in which a vibrating object or system is in resonance with itself. A tone heard from a plucked string is an example of a standing wave in one dimension. Liquid cymatics is about two-dimensional standing waves on a circular membrane (the liquid's surface), much like a vibrating drum head. Click HERE to see standing waves on a vibrating circular membrane, shedding light on the surface of liquid in liquid cymatics.
  6. Mode of Vibration​ - The formal terminology for a standing wave in a vibrating system. Modes of vibration are forms of resonance, and refer to consistent topologies (or landscapes) in the surface of the fluid where certain regions are oscillating up and down maximally and other regions, called nodes, are stationary.
  7. Hydroglyph - The term used to define an image formed by photographing lights reflecting off a liquid system in vibration. Generally speaking, a hydroglyph is any image formed in this way, though typically it is used to describe images of highly-geometric mandala-like standing waves. A hydroglyph image can be thought of as a long-exposure photograph on a short time scale. If the camera is set at 30fps, the shutter is then open for .017 seconds for each photograph. In other words, a hydroglyph is the image of trails of lights as they are reflected off the surface of a moving fluid in .017 seconds if the camera is set at 30fps.
  8. Hydroglyph Cycle - This is the term used to refer to the total repeating pattern seen with the camera. As the frame rate of the camera is altered, one can lock onto elements of this repeating cycle produced by the rhythmic undulations in the fluid below. Since the fluid's surface is distorted, the reflection of the lights from above is also distorted; though because the fluid's distortion is a cyclic mode of vibration the reflection of the lights also encounter a cycle. 
  9. Viscosity - Term used to describe the lack of fluidity of a material. A fluid with a higher viscosity is slow and thick compared to a fluid with a lower viscosity.
  10. Surface Tension - The tension of a surface of a material caused by the attraction/cohesion of the particles of the material in the surface layer. It is because of the high surface tension of water that insects are able to walk and stand on the surface.
  11. Density - The measure of how compact a material is. A fluid that is dense is heavier and more thick than a fluid that is light and runny.

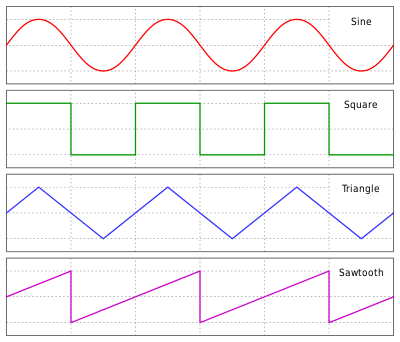
Basic ​Waveforms

​Mode of Vibration

Hydroglyph

The Factors of Liquid Cymatics

  • Input Frequency/Energy - The input frequency, the waveform of the signal, and the amplitude of the signal all play a role in the formation of standing waves. If a particular sine wave frequency excites a fluid system into resonance, changing that same frequency to a sawtooth wave or a square wave, for example, will change the way in which the signal is delivered and thus may change the effect of resonance.
  • Fluid and Cup - The fluid and cup combination create a unique system that has its own fundamental resonant frequency and modes of vibration. Changing the diameter the cup or changing the type of fluid in the cup will each alter the fundamental resonant frequency of the system. Further, changing the shape of the cup from circular to a particular type of polygon will also change the resonant frequency of the cup/fluid system.
  • Illumination - Lighting a standing wave with different forms of lights results in different variations in the way the geometry of the hydroglyph is revealed. The color, brightness, and distance of the lights all play a major role in how we see the topology (or contour) of the surface of the water. The type of the light also affects how we see the reflection: LEDs differ from halogen lamps which also differ from lasers.
  • Observer/Camera​ - A hydroglyph, like a rainbow, is 100% dependent upon the location of the observer (or camera). If the camera lens is moved in any direction, the hydroglyph is altered. Though most hydroglyphs are captured from directly above the vibrated fluid, a lot of important study comes from viewing a standing wave in action from the side. 
  • Level and Balanced - To truly capture the full potential of a two-dimensional liquid standing wave, the cup and speaker combination needs to be level and balanced in a full 360-degree circle (no roll or pitch). If the system of fluid is not fully level and parallel to the ground then the fluid will do what fluids do best and accumulate more towards the low side of the cup which disrupts the potential for the standing wave. Keeping the surface of the fluid (thought of as an elastic membrane) as flat as possible yields the best hydroglyphic results!

Axioms of Liquid Cymatics

  1. The surface of the fluid is an elastic membrane.
  2. The frequency of the up-and-down oscillations of the surface membrane is 1/2 the input frequency (as found in THIS paper).
    This means, for example, if your input frequency is 60Hz and creates a geometric standing wave in the fluid, then the surface membrane is oscillating up and down in its vibrational mode at a frequency of 30Hz.
  3. From #2, we see that if we set our camera frame rate at 1/2 the input frequency we will have a phase-locked image of the hydroglyph.

Theorems of Liquid Cymatics

  1. Any frequency can produce any standing wave geometry if and only if the fluid and container are chosen appropriately.
    Proof: 
     (-->) Assume that a given frequency is capable of producing every possible n-fold geometric standing wave. Given what we know about frequencies, wavelengths, resonance, and basic acoustics, we know that a single frequency can only produce a small, finite, amount of standing waves in a given fluid held in a given pan. Thus, down to the physical limits of the fluid itself and the abilities of the transducer, we can choose pans of theoretically infinitely-small and infinitely-large diameters. Further, by changing the fluid we once more introduce a new spectrum of resonance in the cup/fluid system. Therefore, to achieve any of the infinitely-many possible geometric standing waves a given frequency can produce, we must choose the pan diameter and the fluid properties appropriately to accept that frequency in resonance.
    (<--) Assume that we have a given fluid and a given cup diameter, and that we can analyze the frequency spectrum to identify frequencies that produce vibrational modes in the given system. Modes of vibration are discrete states that cover a small band of frequencies per each mode (usually around .2-.5Hz). That is, each mode of vibration for a given system is achieved by a small window of frequencies. If we were to theoretically analyze all possible fluids in pans of all possible diameters we could analyze the bands that produce a given geometry and see that it will cover all frequencies in the range of study. Because the given geometry is arbitrary, we can then create a chart of frequencies and geometries with the corresponding fluid/pan information that yields that geometry. Therefore, it is possible to find a system for any frequency to produce any standing wave geometry.

Common Misconceptions in Cymatics and Resonance Science

  • "This is what that frequency looks like!" - This is a common statement in many memes and other forms of misinformation spreading around social media. A hydroglyph of a certain frequency only has the geometry it has because that is how that particular frequency interacts with the particular cup and fluid used. If one changes the cup shape or diameter, or the fluid in the cup, the way the frequency interacts in the space will change. As you can see in the images below, 15.6Hz is shown as a 3-fold geometry resonating in a 2"-diameter cup and as a 4-fold geometry resonating in a 2.875"-diameter cup of the same fluid.
  • From understanding the previous fact, we can see that *any* frequency can generate *any* geometry, as long as we choose a cup with an appropriate diameter filled with a fluid with the right surface tension and viscosity. This is the importance of continuing research in studying the vibrational modes of these fluid membranes.

Other Factors that Affect Standing Waves

  • Temperature is a factor in the creation of a liquid standing wave in the sense that temperature alters viscosity. An increase to the viscosity of the fluid corresponds to a decrease in the wave velocity in the fluid and thus a decrease in the resonant frequency of the system. 
  • The depth of the fluid in the container will affect the standing wave as well. First, if the depth is too shallow then the surface of the fluid (thought of as an elastic membrane) cannot flex fully up and down and thus the standing wave cannot be achieved. Conversely, if the depth is too great that it loads too much mass on the speaker then the speaker's ability to perform effectively is drastically reduced. Further, article #11 in the References for Further Study below shares more information on how the depth affects the outcome of a cymatics experiment.
  • The air pressure in the room where the cymatics experiment is taking place matters as well. If there is greater or lesser pressure on the surface of the fluid then we will see the ways in which frequencies affect the surface membrane will be different. A cymatics experiment in a room full of sulfur hexafluoride will  result differently than the same experiment in a vacuum chamber. The surface pressures matter! It is for this reason that altitude also affects the results of a cymatics experiment.
  • Similarly to air pressure, the effects of gravity play a role in the creation of a standing wave. For instance, if we find that a certain frequency creates a 5-fold standing wave in a certain cup, we can take the whole system to the moon and the same system will result differently.
  • Subtle energies can possibly alter the formation of the standing wave in the cymatics experiment. We will soon be experimenting to confirm or deny this possibility.

References for Further Study

  1. CYMATICS - A STUDY OF WAVE PHENOMENA, Hans Jenny, Vol. 1 and 2 (1972).
  2. VIBRATONS OF A CIRCULAR MEMBRANE, Wikipedia.
  3. FARADAY WAVES, Wikipedia.
  4. DETERMINANTS OF FARADAY WAVE-PATTERNS IN WATER SAMPLES OSCILLATED VERTICALLY AT A RANGE OF FREQUENCIES FROM 50HZ TO 200HZ, Sheldrake and Sheldrake, 10.14294/WATER.2017.6, 2017. (2017)
  5. ACOUSTICS AND VIBRATION ANIMATIONS, Dr. Daniel A Russell, Penn State College of Engineering.
  6. VIBRATIONAL MODES OF A CIRCULAR MEMBRANE, Dr. Daniel A Russell, Penn State College of Engineering.
  7. POINT-DRIVEN MODERN CHLADNI FIGURES WITH SYMMETRY BREAKING
    Tuan, Lai, Wen, Huang & Chen (2018); https://www.nature.com/articles/s41598-018-29244-6www.nature.com/articles/s41598-018-29244-6
  8. THE STABILITY OF THE PLANE FREE SURFACE OF A LIQUID IN VERTICAL PERIODIC MOTION
    Benjamin & Ursell (1954); https://cns.gatech.edu/~predrag/GTcourses/PHYS-4421-02/lautrup/BenjUrsell1954.pdf
  9. PARAMETRICALLY FORCED SURFACE WAVES
    Miles & Henderson (1990); https://pdodds.w3.uvm.edu/teaching/courses/2009-08UVM-300/docs/others/1990/miles1990a.pdf
  10. PARAMETRIC INSTABILITY OF THE INTERFACE BETWEEN TWO FLUIDS
    Kumar & Tuckerman (1994); https://blog.espci.fr/laurette/files/2018/01/KT1994JFM279.pdf
  11. PATTERNS AND QUASI-PATTERNS IN THE FARADAY EXPERIMENT
    Edwards & Fauve (1995); https://www.cambridge.org/core/journals/journal-of-fluid-mechanics/article/abs/patterns-and-quasipatterns-in-the-faraday-experiment/8FF2B22DE046BC52D144128237B9C194
  12. PATTERNS AND SPATIOTEMPORAL CHAOS IN PARAMETRICALLY FORCED SURFACE WAVES: A SYSTEMATIC SURVEY AT LARGE ASPECT RATIO
    Kudrolli & Gollub (1996); https://pdodds.w3.uvm.edu/files/papers/others/1996/kudrolli1996b.pdf
  13. THE EFFECT OF DEPTH ON STANDING WAVE PATTERNS
    Binks et al. (1997);  https://pure.tue.nl/ws/files/1433797/Metis132531.pdf
  14. FREQUENCIES THAT GENERATE VARIOUS MODES
    Ciliberto & Gollub (1985);  https://www.cambridge.org/core/journals/journal-of-fluid-mechanics/article/abs/chaotic-mode-competition-in-parametrically-forced-surface-waves/0FAB93EDA61950BC284625B4BF9658C4
  15. Visit our PRODUCTS page to see how we are addressing all of the above factors with our Halo Light System and Sonic Visualizers!
  16. Click ​HERE to visit our Facebook group, "Resonance & Beyond" featuring up-to-date cymatics content from around the world.

Interesting and Insightful Cymatics Videos from Youtube

Cymatics Videos from Resonant Devices

These are some basic videos showing liquid cymatics in action using lights from our Halo Light System; you can read more about our custom device HERE. Further information for each video can be found by visiting the video's page on Youtube and reading the description.